Beginner Intermediate Advanced Cool Topics Reference Search

Games and Fun Stuff Feedback Meeting Forum
Main Page
FizziCalc
Topics
Beginner
Intermediate
Advanced
- Brief Calculus Overview
- Vector Products
- Kinematics with Calculus
- Mechanics with Calculus
- Rotational Mechanics
- - Rotational Inertia
- - Torque
- - Angular Momentum
- Electricity and Magnetism
- - Electric Fields
- - Gauss's Law
- - - Applications
- - Electric Potential
- - Capacitors
- - Kirchhoff's Loop Laws
- - Magnetic Fields
- - - Fields of Currents
- - - Ampere's Law
- - Induced EMF
- - Inductance
Cool Topics
Reference
Features
Customize
Search
Games and Fun Stuff
Meeting Forum
Physics Links

Magnetic Fields

 Back Next
Return to the Fizzics Fizzle Main Page.
Return to the Advanced Level Page.
You have a basic understanding of the magnetic field already but we will review the relationship between it and the electric field and then venture into more involved concepts. It is now known that all magnetic phenomena result from forces between moving electric charges. The work of Hans Christian Oersted (1777-1851) has shown that magnetic effects could be created by moving electric charges. Michael Faraday (1791-1867) and Joseph Henry (1797-1878) showed that currents could be produced by moving magnets.

In describing the interaction of two charges at rest, we introduced the electric field, and described interaction in two stages:
  1. One charge creates an electric field E around it.
  2. The electric field exerts a force F = qE on a charge q placed in the field.
With interactions of charges in motion we get:
  1. A moving charge, or a current, creates a magnetic field around it.
  2. The magnetic field exerts a force on a moving charge or a current in the field.
Now just like an electric field, a magnetic field is a vector field with a vector quantity associated with every point in space. We use the symbol B for the magnetic field (just like we use E in the electric field).

Motion of Charged Particles in Magnetic Fields

You know from your right hand rule that in a magnetic field into the page and a velocity towards the right, a positive charge will be forced upward. But because of this force, its velocity also changes upward. So what results is a positive charge that goes in a circle around and around and around. The magnitude of the force remains the same and it's the direction that changes (always right angle to the velocity) and moves it in a circle.
The force becomes just like a centripetal force. The orbit of the particle is a circle with a constant tangential speed of v. Now you know centripetal acceleration is v2/R so from Newton's second law:
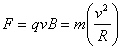
F = qvB = m(v^2/R)
(Equation 6-59)

With some rearranging we find the radius of the circular orbit to be:
R = (mv)/(Bq)
(Equation 6-60)

From the equation you will notice that the radius is proportional to the momentum of the particle (mv) and that the work of the magnetic force is zero because that force is at right angles to the direction of motion. The motion of a charged particle under the force of a magnetic field alone is always motion with constant speed.

Magnetic Force of Conductors with Currents

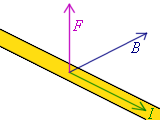Force on a Conductor Illustration When a current-carrying conductor lies in a B field, magnetic forces are exerted on the moving charges. In turn, these forces are transmitted to the conductor. The illustration at right is a representation of part of a conducting wire. We will be considering the force on part of the wire of length L. Charges in the wire will drift past a point in the wire in a time L/vd, carrying a charge of:
q = i(L/v[d])
(Equation 6-61)

Thus:
F = qv[d]B = ILB
(Equation 6-62)

Force and Torque on a Rectangular Loop

Now what if you put an entire circuit into a magnetic field? There will be some amount of force which may even cause torque on the circuit The total force and torque on any conductor can be divided up into segments and applying F = ILB. Usually this requires integration but when the B field is uniform the calculation is a lot simpler.

Rectangular Loop VRML Model Our first example, the rectangular loop, is shown at right (click on the illustration for a VRML model of the rectangular loop). The rectangular loop has sides of length a and b. The normal to the plane of the loop makes an angle a with the direction of a uniform magnetic field. The loop carries current I.

The force is on the right, of length a, and it's in the direction of the x-axis. On this side, B is perpendicular to the current direction and the total force on this side is:
F = IaB

And the same force in the opposite direction also acts on the other side. The forces on the sides of b, represented by F' and -F', are:
F' = IbB cos a

Both forces lie on the y-axis. The total force on the loop is zero, since all the forces cancel. F' and -F have no torque but F and -F do and constitute a couple. The torque of the couple is:
G = (IBa)(b sin a)

According to the equation, and your general knowledge of physics, you know that the torque is greatest when a = 90°, and it's zero when a is 0° or 180°. Since ab is the area A of the loop, we can make that substitution:
G = IBA sin a

The product IA is called the magnetic moment m of the loop. Thus the euqation can be simplified further:
G = mB sin a

Because of the directional relations shown, the torque tends to rotate the loop towards the equilibrium position, which is in the decreasing a, in the plane of the xy-axis perpendicular to the field B.

Force and Torque on a Circular Loop

You can use a large number of small rectangular loops to find the torque, then sum them up so that their areas approach the area of the circular one. It's what calculus was meant for and it's beautiful. What this also means is that you could have a pentagon loop or a weird messed up shape (any shape as long as it's a planar loop) and use this method. But for a circular loop of radius R:
G = pR2IB sin a

Force and Torque on a Solenoid

A solenoid is a helical winding of wire, usually made by winding it around a circular cylinder. If the windings are close, you can just approximate it by circular loops lying in planes at right angles to its axis. So for N turns you easily get:
G = NIAB sin a

Back Next
Created by TQ Team 16600: Clyde Law, Chetan Taralekar, Jim Wang
 E-mail us!
Team Coaches: Melanie Krieger, Chhaya Taralekar