FizziCalc
Intermediate
MechanicsKinematicsForce and EquilibriumEnergy and WorkMomentumGravitationCircular MotionSimple Harmonic MotionElectricity and MagnetismStatic ElectricitySimple CircuitsMagnetismAdvanced
Cool Topics
Reference
Search
Games and Fun Stuff
Meeting Forum
Physics Links
Energy and Work |
 
|
Return to the Intermediate Level Page.
Energy is present in all different forms everywhere. Energy is defined as the capactiy to do work. What is work exactly? Everyone uses the term very loosely. Well the physics definition of work is what is done to an object when a force is applied to move it. In mathematical terms, work is equal to the force times the displacement:
The SI unit for work is the joule (J). One joule of work is doen when a force of one newton causes a displacement of one meter.
The confusing thing about work is that even when a force is applied to a mass, but the mass does not move, no work is done. Even if you were holding up a really heavy object at a constant height, you would be doing no work.
Power
The rate at which work is done is the power. In other words, power is the work done in a certain amount of time:
(Equation 1-8)
Well work is force times displacement, but isn't average velocity displacement over time? Thus:
(Equation 1-9)
The SI unit for power is the watt (W). One watt of power equals one joule of work done in one second. You can also say that one watt is the amount of power that one newton of force applies to move an object with an average velocity of one meter per second.
Potential Energy and Kinetic Energy
There are two main types of energy that we will be discussing: potential energy and kinetic energy. Potential energy is the energy that an object has due to its position or condition. Kinetic energy is the energy that an object has due to its motion.Let's start with potential energy. One type of potential energy, gravitational potential energy, is the work that is done against gravity to move an object up to a certain height above the surface of the earth. If the object falls, the potential energy is lost and is converted into kinetic energy (which is evident as the object increases its velocity as it falls). This energy can do work. For example, falling water from a water fall can be used to turn turbines in a hydroelectric power plant to create electricity.
So how do you calculate gravitational potential energy? Let's start with the general definition of work:
Well, the force is gravity, which is mg. And the displacement is the height. And since the potential energy (usually denoted as U or PE) is the work needed to stay against gravity:
Energy can be stored in springs too, right? This kind of potential energy is called elastic potential energy. The amount that a spring is stretch is directly proportional to the force applied. This relationship is called Hooke's Law:
The k is a spring constant, which varies depending on the type of spring used. x is the displacement of the spring from its original position. Since the force used here is not constant like the other examples (the displacement can change), we can't simply multiply the force by the displacement to find the elastic potential energy. It turns out that the elastic potential energy is:
(Equation 1-10)
Now let's discuss kinetic energy a bit further. Kinetic energy is determined by how fast a mass moves. The equation to find the kinetic energy (usually denoted as K or KE) of a mass moving at a certain velocity (v) is:
(Equation 1-11)
Conservation of Energy
Energy is always conserved. This is called the law of conservation of energy. For example, if you lose potential energy, you must gain some other type of energy, like kinetic energy for example. The total energy you have at the beginning equals the amount you have at the end.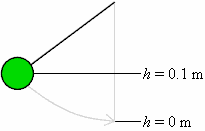
Okay, so what can you use this for?
Let's use a ball hanging on a string (basically like a pendulum) for an example.
The ball weighs 1 kg.
We will take the ball and move it out until it is 0.1 m above its lowest point (see illustration at right).
Now, if we let the ball go, it will swing downward.
What will its velocity be when it passes its lowest point?At first, this may not seem like a problem related to energy, but it is. What is the total energy of the ball when it is at 0.1 m (we will call this point 1)? Well, the kinetic energy is 0 since it is initially at rest up there. However, the potential energy is:
Now let's look at the bottom of its path (we will call this point 2). At this point the potential energy is 0 since its height is 0. However, the kinetic energy is:
(Equation 1-12)
Using the law of conservation of energy, we can say that the total energy at point 1 equals the total energy at point 2. Since there is only potential energy at point 1 and kinetic energy at point 2, we can equate them and solve for the velocity at point 2:
U1 = K2
0.98 J = (0.5 kg)v22
1.96 m2/s2 = v22
v2 = 1.4 m/s
Therefore, the ball will swing past the lowest point with a velocity of 1.4 m/s.
Clyde Law, Chetan Taralekar, Jim WangMelanie Krieger, Chhaya Taralekar