FizziCalc
Intermediate
MechanicsKinematicsForce and EquilibriumEnergy and WorkMomentumGravitationCircular MotionSimple Harmonic MotionElectricity and MagnetismStatic ElectricitySimple CircuitsMagnetismAdvanced
Cool Topics
Reference
Search
Games and Fun Stuff
Meeting Forum
Physics Links
Simple Harmonic Motion |
 
|
Return to the Intermediate Level Page.
An object is said to be in simple harmonic motion if the following occurs:
- It moves in a straight line.
- A variable force acts on it.
- The magnitude of force is proportional to the displacement of the mass.
- The force is always opposite in direction to the displacement direction.
- The motion is repetitive and a round trip, back and forth, is always made in equal time periods.
Springs
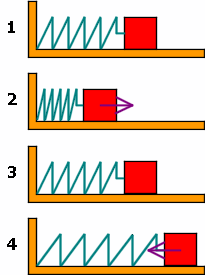A spring that is oscillates an attached mass back and forth on a frictionless surface is an example of simple harmonic motion.
The magnitude and direction of the force in terms of its displacement is:k is a constant for the particular spring and x is the displacement. The right side of the equation is negative because the force always acts opposite the displacement (positions 2 and 4 on the illustration at right). No force is acting when the mass returns to its original position (positions 1 and 3), but the mass keeps moving because it was moving before. In addition, at the original position, the mass is moving at its highest speed. Once it passes the original position, the force acts to slow it down and move it in the opposite direction. The entire cycle repeats again and again in a certain period of time.
The period (T) of one full cycle can be calculated with the following equation:
(Equation 1-21)
If you look at the equation for the period, you'll notice that it is independent of the maximum displacement. No matter how far you stretch or compress the spring, the period will remain the same.
Pendulums
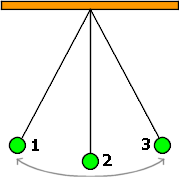The motion of a pendulum can be considered simple harmonic motion even though the bob hanging at the end of the string moves in a curve.
This is because if the string is relatively long compared to the initial displacement, the curve made by the bob is close enough to a straight line.The pendulum works almost like the spring. The force is always pointing opposite the displacement. The bob is moving the fastest when it passes its lowest point (position 2 on the illustration at right). The pendulum continuously moves back and forth. Also, the equation for the period of a pendulum is similar to that of the spring:
(Equation 1-22)
L is the length of the string and g is the gravitation acceleration (your good ol' 9.8 m/s2). Note that the period does not depend at all on the mass or the initial displacement.
Check out the Pendulum in our games and fun stuff section to experiment with how a pendulum works.
Clyde Law, Chetan Taralekar, Jim WangMelanie Krieger, Chhaya Taralekar