FizziCalc
Intermediate
MechanicsKinematicsForce and EquilibriumEnergy and WorkMomentumGravitationCircular MotionSimple Harmonic MotionElectricity and MagnetismStatic ElectricitySimple CircuitsMagnetismAdvanced
Cool Topics
Reference
Search
Games and Fun Stuff
Meeting Forum
Physics Links
Force and Equilibrium |
 
|
Return to the Intermediate Level Page.
It's all good if you can tell where something is going to move, but what about how it starts moving in the first place? Well, according to Newton's Second Law of Motion, to move an object, you have to apply a force. The larger the object, the larger the force you need to apply. In fact, the relationship is actually really simple:
The equation states that force is equal to the mass times the acceleration. In other words, a certain amount of force will accelerate a certain amount of mass.
Force is also a vector quantity. For example, if you push a box with a force of 8 N to the right while your friend pushes with a force of 5 N to the left, it would be as if one person was pushing with a force of 3 N to the right.
Well, what kind of forces are there? Well, the kinds that you probably will be working with most is weight and friction.
Weight
The force that causes an object to fall due to gravity is called the weight of an object. On the surface of the earth, the acceleration of an object due to gravity is about 9.8 m/s2. This is usually refered to as g. So, we can write the equation we stated before in a little different format to specify weight:The subscript W is used to denote that it is the weight force. Also notice that we replaced the a with g.
So what about an object sitting on a table? It is not falling because the table is holding it up, right? The object is said to be at equilibrium because it is not being accelerated. The net force on the object is 0. Okay, there is the weight force pulling the object down, so something must push it up to make the net force 0. This force is called the normal force. The normal force (FN) is always exerted perpendicular to the surface that is holding up the object, in this case the table.
For a level surface, the magnitude of the normal force is equal to the magnitude of the weight force, except that it acts in the opposite direction.
But what about for a slanted surface like a ramp?
Well, look at the illustration to the right.
The left part of the illustration shows what happens on a level surface.
The right part shows what happens on a slanted surface.Notice how the normal force is still perpendicular to the slanted surface. It cancels out the component of the weight force perpendicular to the slanted surface (shown by the dotted line perpendicular to the slanted surface). But it does not cancel out the weight force entirely. So what is left is the component of the weight force parallel to the slanted surface (shown by the dotted line parallel to the slanted surface). This is called the net force (Fnet). As you can see, the net force is directed towards the bottom of the ramp. This is why the block will slide down the ramp.
Let's put this in more mathematical terms so that you can figure out actually what the magnitude of the net force is. Say we have a ramp at an angle of q. The magnitude of the normal force is equal to the magnitude of the component of the weight force perpendicular to the slanted surface. So what is the magnitude? Well, through simple trigonometry, the magnitude of the normal force is:
By the same reasoning, the magnitude of the net force is:
Notice what happens when the q equals 0 (a level surface). Since cos 0 = 1 and sin 0 = 0, the equations merely simplify to:
and
Fnet = 0
...which is what we would expect.
Friction
All this time we have been ignoring friction, but friction plays a big role as well. Friction is a force that acts parallel to the surfaces in contact, and it turns out that it is determined by the normal force. The ratio of the frictional force to the normal force called the coefficient of friction (denoted by the Greek letter, m). To find the force of friction (Ffr):There are two types of friction that we will be dealing with, static friction and kinetic friction. Static friction is the friction between two surfaces at rest. To start moving an object, you have to overcome the force of static friction. Kinetic friction is the friction between two surfaces that are moving with respect to one another. To keep an object moving, you have to overcome the force of kinetic friction. Generally the force of static friction is greater than the force of kinetic friction. Thus, the coefficient of static friction (ms) is greater than the coefficient of kinetic friction (mk). The coefficients vary depending on the materials in contact.
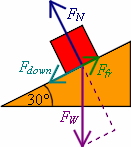Okay, let's put everything you just learned into one big example.
We have a 5 kg block sitting on a ramp at an incline of 30°.
This time friction is acting.
The coefficient of static friction between the block and the surface is 0.5.
The coefficient of kinetic friction is 0.2.
Will the block begin to slide?
If so, how fast does it accelerate down the ramp once it begins to slide?It may look complicated at first but it really isn't too bad if you take it one step at a time. Let's first find the weight force:
Now, let's find the force that makes the block move down the ramp. This time we do not call it Fnet since it doesn't take all the forces into account (we're leaving out friction). Instead, let's call it Fdown:
Now let's find the force of friction. But since the force of friction depends on the normal force, we have to find the normal force first:
Okay, now that we have the normal force, which coefficient of friction do we use? Well, we're trying to find out whether the block will begin sliding, so we should use the coefficient of static friction:
Since the friction is acting directly opposite of the down force, we merely subtract the down force and the friction to find the net force:
Now we can say for sure that the block will slide down the ramp because the net force is positive (Fdown is greater than Ffrs).
What about the next part of the question? Since the block is moving now, we should find the force of kinetic friction:
And then we should find the new net force with that force of friction:
Now we can plug this answer back into the general definition of a force to find the acceleration:
16.0 N = (5 kg)a
a = 3.2 m/s2
And there you have it! The block accelerates down the ramp at 3.2 m/s2.
Check out the Vector Analyzer in our games and fun stuff section to see how vector forces interact to move a body.
Clyde Law, Chetan Taralekar, Jim WangMelanie Krieger, Chhaya Taralekar