FizziCalc
Intermediate
MechanicsKinematicsForce and EquilibriumEnergy and WorkMomentumGravitationCircular MotionSimple Harmonic MotionElectricity and MagnetismStatic ElectricitySimple CircuitsMagnetismAdvanced
Cool Topics
Reference
Search
Games and Fun Stuff
Meeting Forum
Physics Links
Momentum |
 
|
Return to the Intermediate Level Page.
Every moving object has momentum. Momentum (denoted as p) is just the product of the mass and velocity:
If a force is applied to an object, the product of the force and the time during which the force was applied is called the impulse. Impulse (denoted at J) is given by the equation:
Now, force is mass times acceleration. Isn't the change in velocity acceleration times the change in time? Thus:
(Equation 1-13)
So impulse turns out to be the change in momentum. For example, if you throw an egg into a brick wall, the egg will crack apart. However, if you throw an egg into a blanket, the egg will stop without breaking. This is because the force needed to produce the necessary impulse to stop the egg is lower since you are allowing more time for the egg to stop by using a blanket.
Conservation of Momentum
The law of conservation of momentum dictates that the momentum of a system of bodies must remain constant if they are not acted upon by outside forces. What does this mean? Well, we can use an example to demonstrate the conservation of momentum. Let's say ball 1 weighs 2 kg and ball 2 weighs 3 kg. Ball 1 is moving to the right toward ball 2 at 2 m/s. Ball 2 is at rest. When they hit, ball 2 moves to the right at 1 m/s. How fast does ball 1 move?To figure this one out, we should find the total momentum of the system before the collision. The momentum of ball 2 before the collision is 0 since it is at rest. The momentum of ball 1 before the collision is:
Now we have to find the momentum after the system. The momentum of ball 2 after the collision is:
The momentum of ball 1 after the collision is:
Now lets set the momentum before equal to the momentum after:
1 kg m/s = (2 kg)vafter1
vafter1 = 0.5 m/s
And we find that the velocity of ball 1 after the collision is 0.5 m/s.
Elastic and Inelastic Collisions
A collision is said to be elastic if the kinetic energy before the collision equals the kinetic energy after the collision. Otherwise, the collision is said to be inelastic. Let's use the example above and test to see if it is an elastic collision.Before the collision, ball 2 has no kinetic energy since it is at rest, but ball 1 has kinetic energy:
(Equation 1-14)
After the collision, the kinetic energy of ball 1 and 2 are:
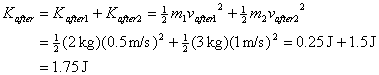
(Equation 1-15)
As you can see, the kinetic energy after the collision is less than the kinetic energy before. Therefore, the collision is inelastic.
But wait a minute! Isn't energy conserved? Yes, energy is conserved, but kinetic energy does not have to be conserved. The kinetic energy that was lost after the collision could have been transferred to other types of energy in the form of heat or sound.
Clyde Law, Chetan Taralekar, Jim WangMelanie Krieger, Chhaya Taralekar